I mentioned that one of the tests – all solitaire – I ran to try out the game and rules was a three-ship setup of the solar system “grand tour” scenario. One of the ships started on Ganymede, and a photo I’d included showed the course.

Here’s an explanation of the course. In retrospect, i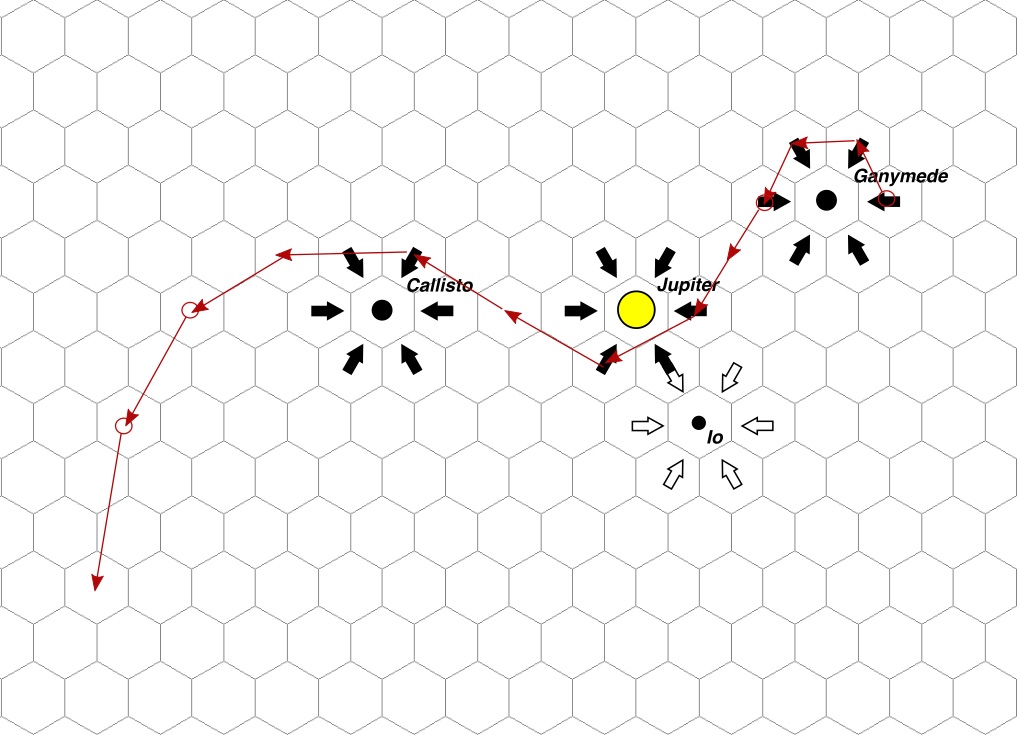](GHOST_URL/content/images/2018/06/triplanetary-course-final.jpg)

Above is the overall course from the beginning through turn 13, as the corvette begins to make speed to shoot a gap in the asteroid belt on its way to bypass Mars. A requirement was that you had to go through the gravity zone of every major body in the system.

The first turn (below image) was launch. Per rules, on a set of boosters with no internal expenditure of fuel, but, leaving the racer in space. Without thrust the next turn, the ship would be pulled back down.

Gravity takes effect the turn after you cross through or enter the hex. So turn 02, gravity (blue arrow in the diagram) would pull the ship back, and the ship thrusts (green arrow) one hex at such an angle as to go into a counterclockwise orbit. Note – unless you overdrive your engines (only warships can), you only get one fuel/hex of thrust per turn. As a result, you generally can’t simply power your way straight out but must at least begin an orbit.

The end result is that I mark a circle for thrust, and draw an arrow from the center of the origin hex to the center 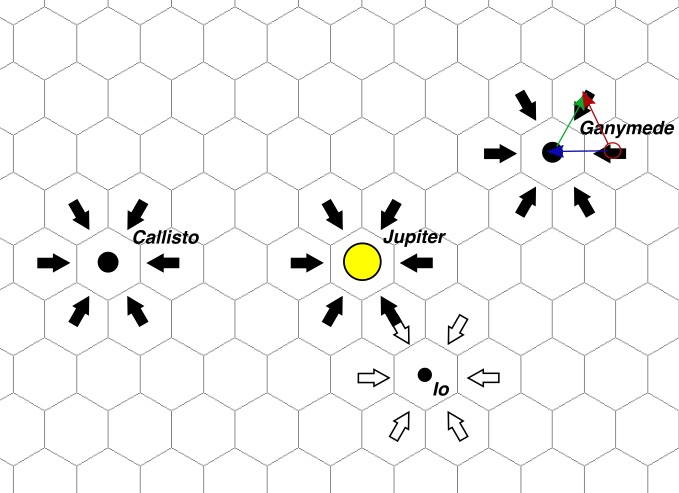](GHOST_URL/content/images/2018/06/triplanetary-course-01-02.jpg)

The third and fourth turns were straightforward. No thrust was applied. The yellow arrow shows the path the ship would have taken by inertia without other forces acting upon it, the blue arrow of the effects of gravity, and the red arrow the final resulting vector. As I mentioned in my last post, yes, we’re in stable orbit, with no additional hi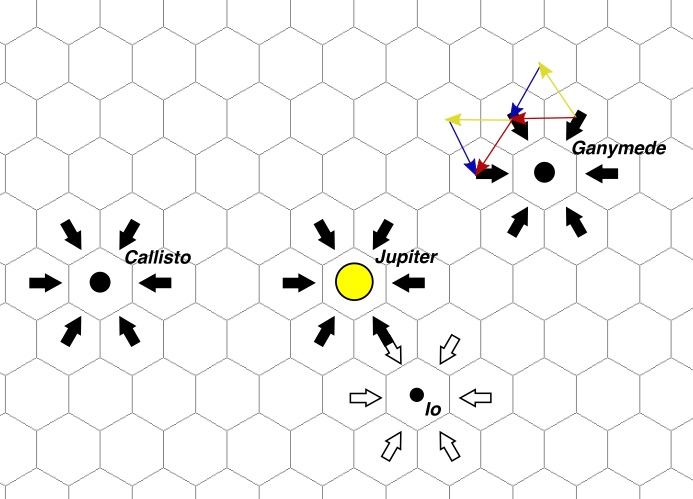](GHOST_URL/content/images/2018/06/triplanetary-course-03-04.jpg)

The ship breaks orbit. Inertia would carry it out but for gravity pulling it around the planet, but this time we apply 1G of thrust and the resulting vector takes us to Jupiter. I’ve marked the board for thrust (and started marking dotted lines for the previous turns course to make it easier to see which gravity effects are in play. In game, you keep at least several turns of course on the board for fuel/etc. tracking. In this case, we were already affected by the down-right arrow the previous turn, and are only due to be affected 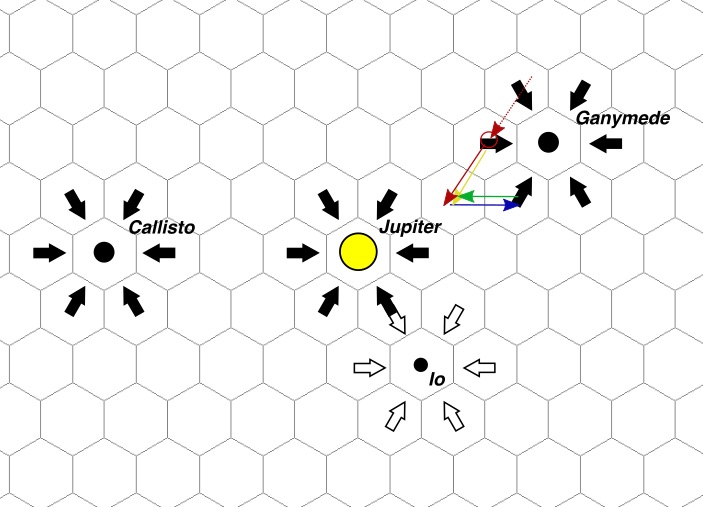](GHOST_URL/content/images/2018/06/triplanetary-course-05.jpg)

No thrust or gravity, we insert into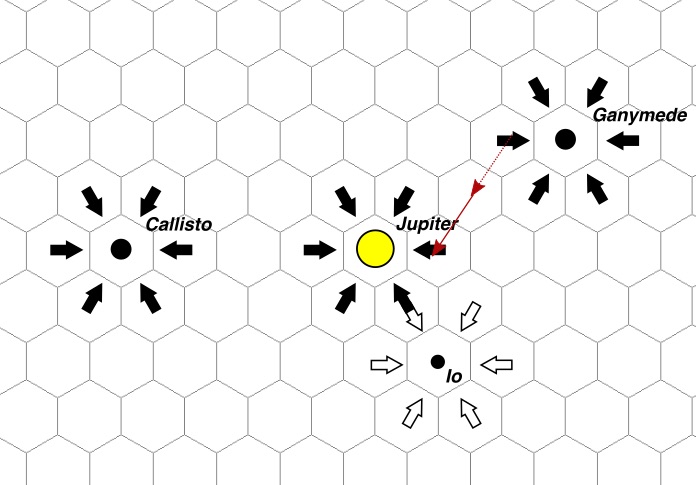](GHOST_URL/content/images/2018/06/triplanetary-course-06.jpg)

It gets fun here. Again, no thrust so far except launch and breaking Ganymede orbit – this is a finesse play. The previous vector would take us between Jupiter and Io, but gravity from the hex entered/passed through pulls us left, speeding us up slightly. We avoid crashing into Jupiter – the line doesn’t actually cross the image of the planet – and for reference will be affected by two grav arrows next turn – the hex we passed inside of/along the inside edge of, as well](GHOST_URL/content/images/2018/06/triplanetary-course-07.jpg)

Plotting our previous (dotted red) vector out along the new projected (yellow) course, the two gravity arrows in effect pull us up.

In case you’re wondering, we avoid being affected by Io due to to separate rules. First, outer hex-sides are not subject to gravity effects. Second, note the hollow arrows. That’s a “weak” gravity field and one can ignore or accept  the effects of the first such hex one passes through. As a result, even if I hadn’t skirted outside the effects any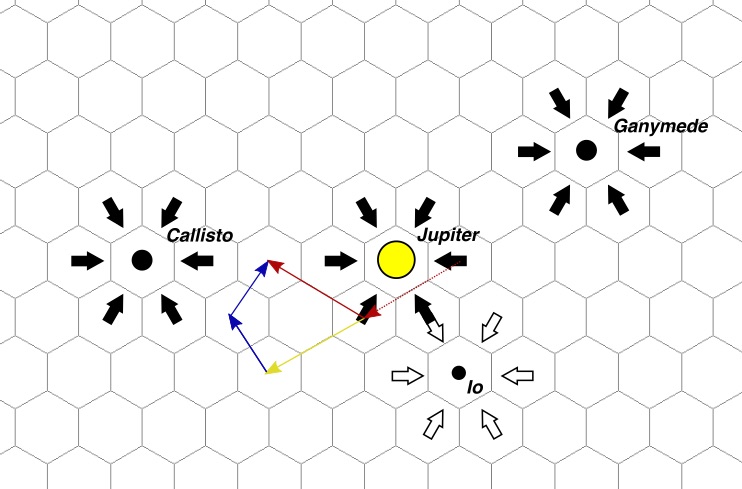](GHOST_URL/content/images/2018/06/triplanetary-course-08.jpg)

With gravity already accounted for in my previous course, and no thrust, I skirt the outer edge of a gravity hex, and enter the gravit](GHOST_URL/content/images/2018/06/triplanetary-course-09.jpg)

Plotting my inertial (yellow) course out plus the gravity (blue) of the hex I entered, My course bends down, to be affected by gravity again next turn, but otherwise esca](GHOST_URL/content/images/2018/06/triplanetary-course-10.jpg)

With the down-right pull as I exit Callisto, my course bends further toward the inner plane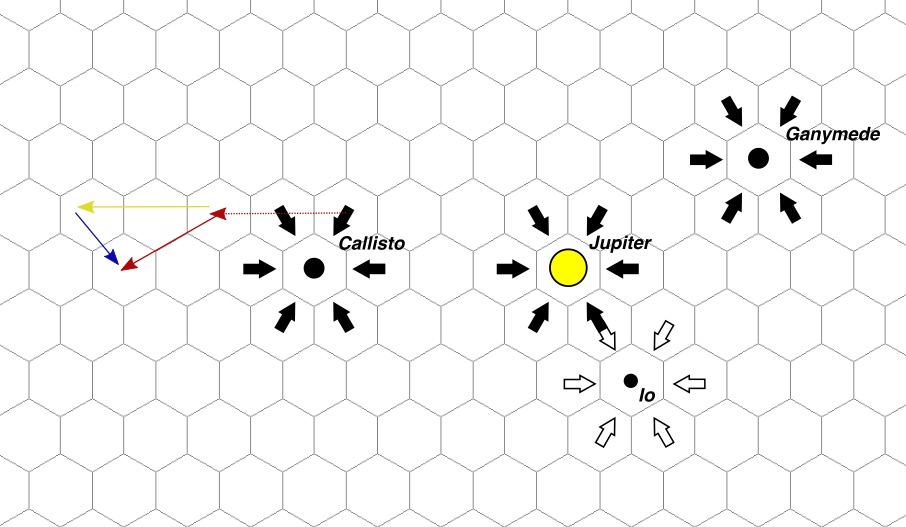](GHOST_URL/content/images/2018/06/triplanetary-course-11.jpg)

Finally I apply thrust to my inertial course and mark a thrust expenditure circle, shifting my course to aim for a gap in t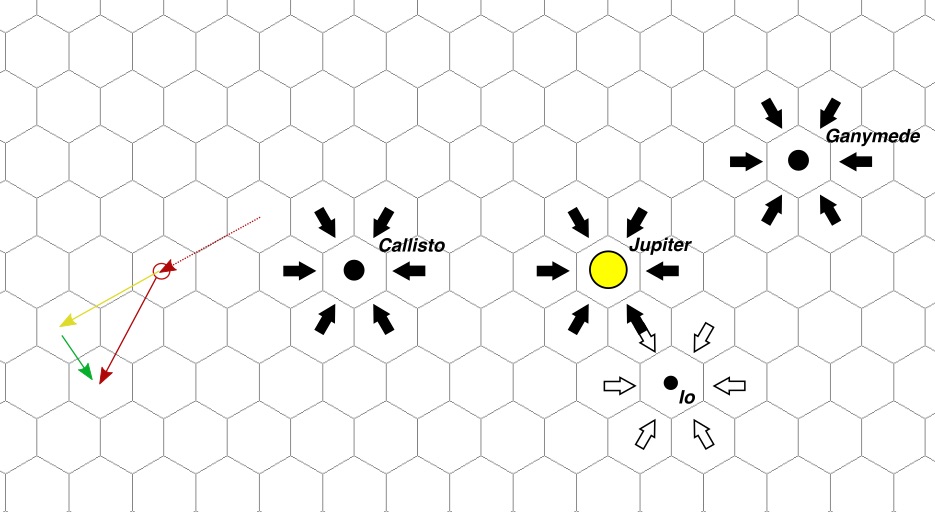](GHOST_URL/content/images/2018/06/triplanetary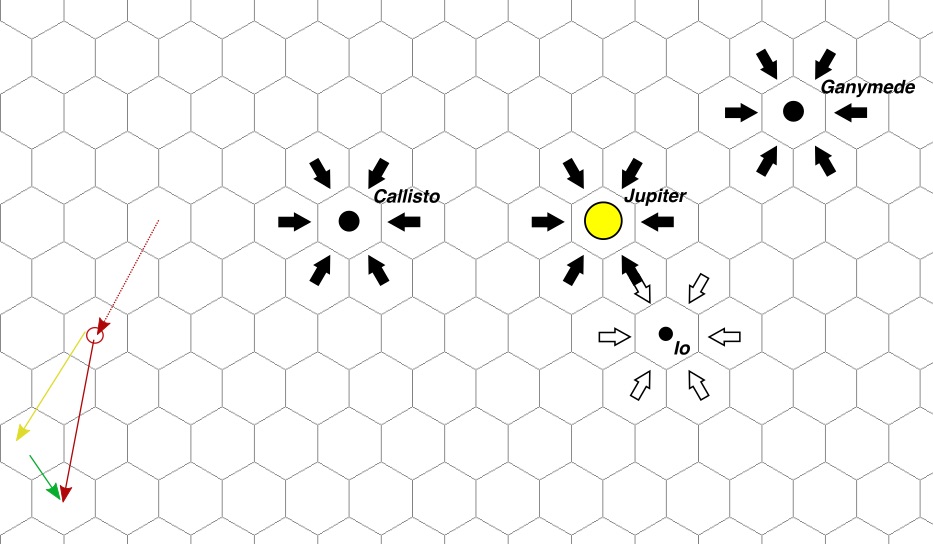](GHOST_URL/content/images/2018/06/triplanetary-course-13.jpg)

There you have it. Not including the free boost to orbital space I threaded three of the planets I had to approach with a mere two points of fuel, and began to make speed for Mars as I fell in toward the inner planets. As noted previously, the terran-origin ship going clockwise around the board overshot at Mercury and had to double back around its own course instead of taking the most efficient path, such that with the need to refuel due to wasted reaction mass and delta-vee,  it and the Ganymede racer crossed paths at Venus. The Venusian racer had just gotten to Terra but had burned enough fuel that it stopped to refuel at Terra before heading out to the Jovian systems. The Ganymede racer is only roughly 15 turns behind the Venusian racer despite taking 22](GHOST_URL/content/images/2018/06/IMG_0901.jpg)